I have seen a few different methods for ratio change problems. Some people use scaling (not pictured), I have often in the past preferred the method in the first picture, forming an algebraic equation, writing as fractions using a part-part comparison, and then solving.
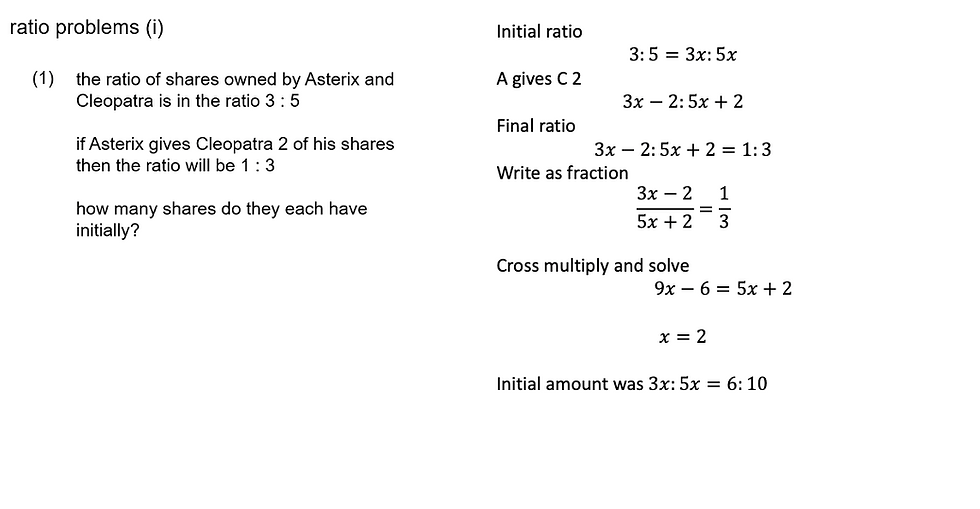
My sticking point was around the writing as fractions, pupils were not familiar enough with the idea of writing fractions in this way (part-part). This could obviously be addressed with a bigger focus on this representation in younger years, but let us just assume that isn't the case, then there are other options.
This option was shown to me by @danicquinn at a La Salle Teach together. I like how it related more to how students normally deal with ratios and fractions, a part-whole relationship.
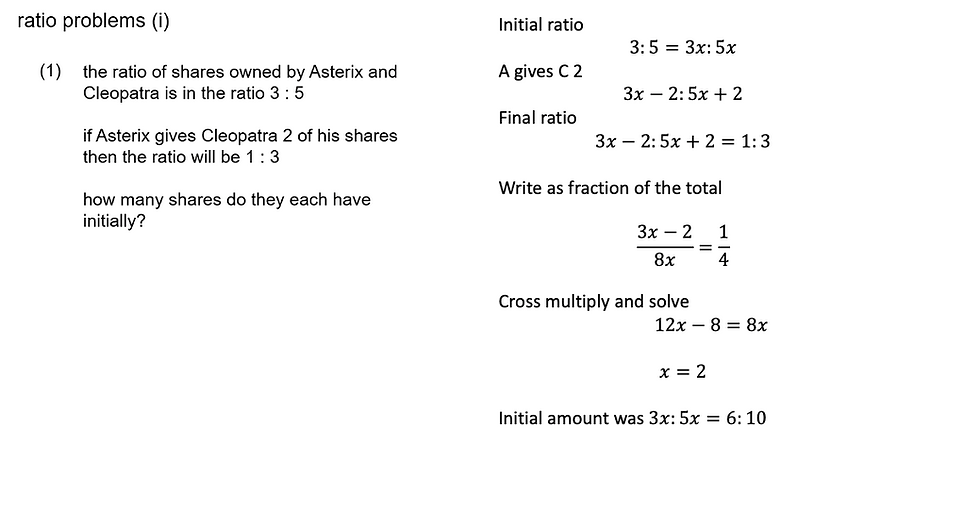
The final option I haven't seen before, and I came up with a tutee, who was struggling with a similar question. We had just done simultaneous equations and it occurred to me to link the two, if we can write the first ratio algebraically then we could also do this with the second.
It is likely that I most certainly am not the first to use this method, and I apologies if it is very obvious, but given the fact I hadn't seen it before despite all my time spent on Twitter it may not be known to some people. I thought it was a really cool link so I wanted to share it.
What do you think? Have you seen this method before, do you like it or use it? What advantages and disadvantages does it have? If you haven't used it before, try it on a few questions and let me know what you think.
The question is taken from the wonderful Don Steward.
Comments