Here is a possible task to introduce sine and cosine to students using the unit circle.
When introducing trigonometry in right-angled triangles students have to combine so many ideas, several of which are novel, which means that it will have a high level of element interactivity and poses a high intrinsic load. One way to overcome the intrinsic load is to atomise the teaching, but I think there is something else we can do to increase students' chances of success, and that is to introduce some of these novel ideas earlier, specifically, the trigonometric ratios.
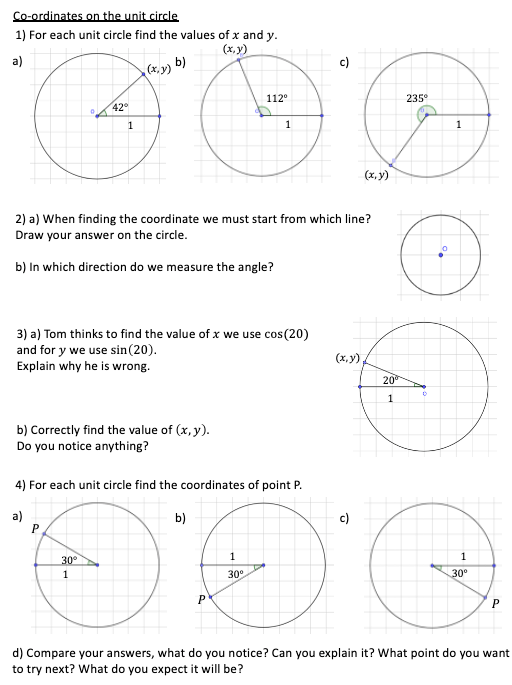
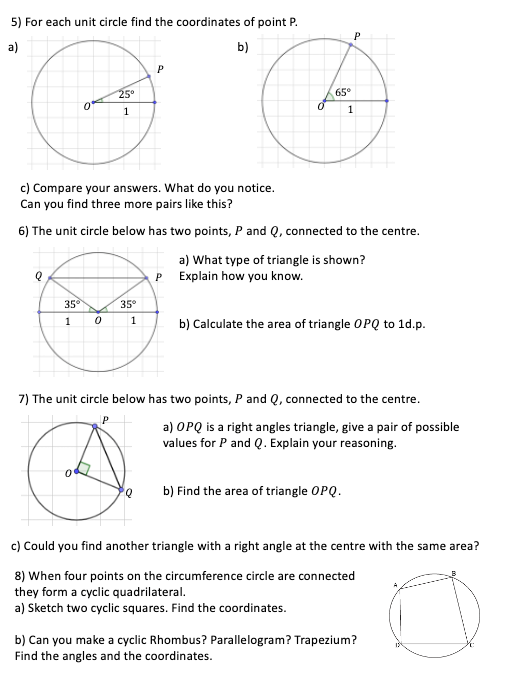
The approach outlined in Dave Hewitt's article Canonical images is incredibly intuitive and I think completely accessible by first and second-year secondary school students, sine represents the height of a coordinate on a unit circle, and cosine the horizontal position. I hypothesis that spending two or three lessons working with the unit circle at least a year before looking at trigonometry in right-angled triangles, it would have reduced the number of novel ideas.
Doing this earlier allows for the maturation of these ideas, and gives students time to make sense of them. Trigonometry could then be built up from an understanding of similarity, by connecting the unit circle to a unit triangle, with a hypotenuse one, and scaling the triangle (a ratio table works really well here).
I think laying the groundwork or planting the seeds of an idea early can be incredibly effective, at least from my experience. There have been times where my classes have really struggled with something, then when we have revisited it the following year, or after an assessment, or even after a holiday period, they pick it up almost instantly. I think it can often be important to consider what could we introduce earlier, or plant the seed of, to help students be successful in the future.
Comments